Complex Numbers and the Stable Characteristic Function.

Where z is complex and r,  θ, u, and v are Real, z can be represented in polar and Cartesian forms:

Expanding the polar form.

Therefore,

Absolute values

Argument

Conjugate

The stable characteristic function, 1 - parameterization

This can be rewritten in polar complex form

Thus

The Quick approximation of stable γ, using empirical characteristic function, ecf, where is each random variable uses the absolute value of the characteristic function.

If is from a stable distribution then

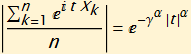
Letting t = 1,

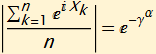
Since stable distributions are scalable, if we divide each by γ the scaled stable characteristic function will have γ = 1 and

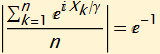
In Mathematica this can be rewritten and solved numerically:

Thus γ can be approximated numerically from the empirical characteristic function independently of α for any stable distribution including the normal distribution.  This is how we approximate market volatility, using a small enough sample over which γ hasn't changed much.

© Copyright 2009 mathestate    Sat 21 Feb 2009