The "Stable" Approach to Data
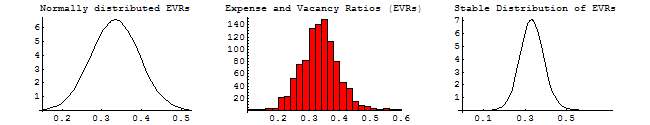
The assumption of normality imposes a set of strong conditions on the data. Included in these are symmetry, thin tails and a finite variance. Relaxing the normality assumption permits a better view of the data and, hopefully, the world from which it is drawn. The normal distribution is a special case of the family of Stable-Paretian (SP) distributions. SP distributions are characterized by four (4) parameters. One of these is alpha, the index of stability, which provides a measure of tail behavior. Another is beta, a skewness parameter. For the (special) normal case alpha = 2 and beta = 0
Because of recent technological developments, we can now estimate Stable parameters. For our data alpha is less than 2 and beta is positive, meaning that it has a heavy right tail. This means that there is more probability in the right (higher in this case) end of the distribution.Notice the similarity in the shape of the histogram of the actual data and the shape of a plot of the Stable pdf on the right. Contrast that with the assumption of normality made earlier as shown in the left plot. The assumption of normality distorts our view of the data away from its actual shape. Note below how the shape of the SP distribution on the right matches the histogram of the data better than the normal distribution does.