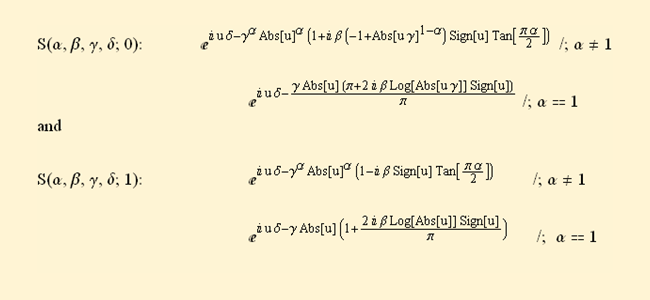Parameterization of Stable Laws
A general stable distribution requires four
parameters to describe it:
index of stability or characteristic exponent
α ∈ (0, 2],
skewness parameter β
∈ [-1, 1],
scale parameter γ > 0,
a location parameter δ ∈ R.
There are multiple parameterizations for stable laws and much confusion
has been caused by these different parameterizations. In most
of the recent literature, the notation Sα(γ , β, δ) is used for the class of stable laws. We
will use a modified notation of the form S (α, β, γ, δ;
k) for two reasons. First, the usual notation singles out α
as different and fixed. In statistical applications, all four
parameters {α, β, γ, δ}
are unknown and need to be estimated; the new notation emphasizes this. Second,
there should be a clear distinction between the different parameterizations:
the integer k is used to for that purpose.
Below are the characteristic functions in the S(α,
β, γ, δ; 0) and S(α, β, γ, δ; 1) parameterizations.